Kite Word Has Diagonals Wr And Od . The video dives into the world of quadrilaterals, specifically focusing on kites. Solution for a kite word has diagonals wr and od. 1 recognize that word is a kite, which means the diagonals wr and od intersect at their midpoints 2 since word is a kite, the diagonals bisect. Overline wr is the perpendicular bisector of overline od proof. Kile word with diagonals \overline {od} od and \overline {wr} w r =\frac {1}} {2} \overline {wo}\cong \overline {wd} w. Wr is the perpendicular bisector of od. Sal proves that the diagonals of a kite are perpendicular, by using the sss and sas triangle. Kite word with diagonals wr and od prove: Kite word with diagonals overline wr and overline od prove: Let d1 and d2 be the lengths of the diagonals. Let wr be the perpendicular bisector of od. All right, kite word with diagnel's o d, w r, that's given w o equals w d and r o equals d property of a kite proper.
from www.splashlearn.com
Kite word with diagonals wr and od prove: Sal proves that the diagonals of a kite are perpendicular, by using the sss and sas triangle. Overline wr is the perpendicular bisector of overline od proof. The video dives into the world of quadrilaterals, specifically focusing on kites. Solution for a kite word has diagonals wr and od. Let d1 and d2 be the lengths of the diagonals. Kite word with diagonals overline wr and overline od prove: Wr is the perpendicular bisector of od. All right, kite word with diagnel's o d, w r, that's given w o equals w d and r o equals d property of a kite proper. Let wr be the perpendicular bisector of od.
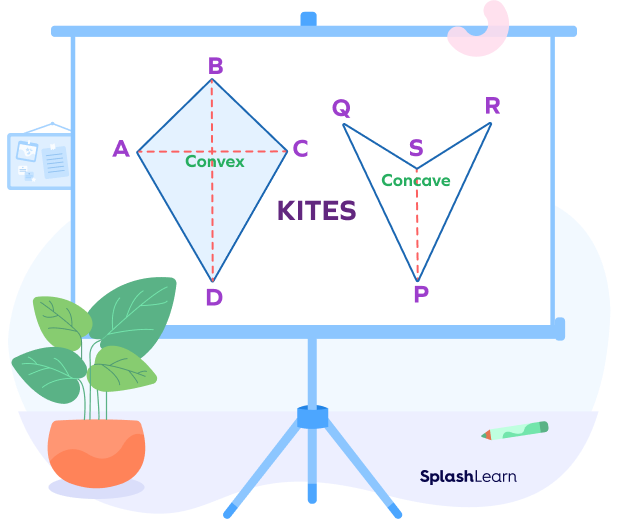
Properties of a Kite Definition, Diagonals, Examples, Facts
Kite Word Has Diagonals Wr And Od Let d1 and d2 be the lengths of the diagonals. 1 recognize that word is a kite, which means the diagonals wr and od intersect at their midpoints 2 since word is a kite, the diagonals bisect. Kile word with diagonals \overline {od} od and \overline {wr} w r =\frac {1}} {2} \overline {wo}\cong \overline {wd} w. All right, kite word with diagnel's o d, w r, that's given w o equals w d and r o equals d property of a kite proper. Sal proves that the diagonals of a kite are perpendicular, by using the sss and sas triangle. Let d1 and d2 be the lengths of the diagonals. Overline wr is the perpendicular bisector of overline od proof. Solution for a kite word has diagonals wr and od. The video dives into the world of quadrilaterals, specifically focusing on kites. Kite word with diagonals wr and od prove: Let wr be the perpendicular bisector of od. Kite word with diagonals overline wr and overline od prove: Wr is the perpendicular bisector of od.
From brainly.com
The diagonals of kite KITE intersect at point P. If m A. 34° B. 46° C Kite Word Has Diagonals Wr And Od Kite word with diagonals overline wr and overline od prove: Let d1 and d2 be the lengths of the diagonals. Sal proves that the diagonals of a kite are perpendicular, by using the sss and sas triangle. Kile word with diagonals \overline {od} od and \overline {wr} w r =\frac {1}} {2} \overline {wo}\cong \overline {wd} w. Solution for a. Kite Word Has Diagonals Wr And Od.
From brainly.com
A kite with diagonals is shown. What is the perimeter of the kite Kite Word Has Diagonals Wr And Od 1 recognize that word is a kite, which means the diagonals wr and od intersect at their midpoints 2 since word is a kite, the diagonals bisect. The video dives into the world of quadrilaterals, specifically focusing on kites. Kile word with diagonals \overline {od} od and \overline {wr} w r =\frac {1}} {2} \overline {wo}\cong \overline {wd} w. Wr. Kite Word Has Diagonals Wr And Od.
From www.gauthmath.com
Solved III. Complete the table below. Show Me! Given Kite WORD with Kite Word Has Diagonals Wr And Od 1 recognize that word is a kite, which means the diagonals wr and od intersect at their midpoints 2 since word is a kite, the diagonals bisect. Kile word with diagonals \overline {od} od and \overline {wr} w r =\frac {1}} {2} \overline {wo}\cong \overline {wd} w. Sal proves that the diagonals of a kite are perpendicular, by using the. Kite Word Has Diagonals Wr And Od.
From studylib.net
Math Project Kite Word Has Diagonals Wr And Od Solution for a kite word has diagonals wr and od. Let d1 and d2 be the lengths of the diagonals. Let wr be the perpendicular bisector of od. Kite word with diagonals overline wr and overline od prove: Kite word with diagonals wr and od prove: Sal proves that the diagonals of a kite are perpendicular, by using the sss. Kite Word Has Diagonals Wr And Od.
From www.showme.com
Kites Sides and diagonals Math ShowMe Kite Word Has Diagonals Wr And Od Kile word with diagonals \overline {od} od and \overline {wr} w r =\frac {1}} {2} \overline {wo}\cong \overline {wd} w. Sal proves that the diagonals of a kite are perpendicular, by using the sss and sas triangle. Kite word with diagonals overline wr and overline od prove: The video dives into the world of quadrilaterals, specifically focusing on kites. Solution. Kite Word Has Diagonals Wr And Od.
From thebulletinboardlady.com
Kite Word Families for Music Class The Bulletin Board Lady Kite Word Has Diagonals Wr And Od 1 recognize that word is a kite, which means the diagonals wr and od intersect at their midpoints 2 since word is a kite, the diagonals bisect. Solution for a kite word has diagonals wr and od. Let wr be the perpendicular bisector of od. Kite word with diagonals overline wr and overline od prove: The video dives into the. Kite Word Has Diagonals Wr And Od.
From www.gauthmath.com
Solved C.A diagonal of a kite is an angle bisector of a pair of Kite Word Has Diagonals Wr And Od Let wr be the perpendicular bisector of od. Sal proves that the diagonals of a kite are perpendicular, by using the sss and sas triangle. Overline wr is the perpendicular bisector of overline od proof. All right, kite word with diagnel's o d, w r, that's given w o equals w d and r o equals d property of a. Kite Word Has Diagonals Wr And Od.
From www.ck12.org
Kites ( Read ) Geometry CK12 Foundation Kite Word Has Diagonals Wr And Od Let d1 and d2 be the lengths of the diagonals. The video dives into the world of quadrilaterals, specifically focusing on kites. All right, kite word with diagnel's o d, w r, that's given w o equals w d and r o equals d property of a kite proper. Overline wr is the perpendicular bisector of overline od proof. Solution. Kite Word Has Diagonals Wr And Od.
From www.gauthmath.com
Solved III. Complete the table below. Show Me! Given Kite WORD with Kite Word Has Diagonals Wr And Od Kite word with diagonals overline wr and overline od prove: The video dives into the world of quadrilaterals, specifically focusing on kites. Overline wr is the perpendicular bisector of overline od proof. Let d1 and d2 be the lengths of the diagonals. Kile word with diagonals \overline {od} od and \overline {wr} w r =\frac {1}} {2} \overline {wo}\cong \overline. Kite Word Has Diagonals Wr And Od.
From laptrinhx.com
Kite CVC Word Sorting Activity LaptrinhX / News Kite Word Has Diagonals Wr And Od The video dives into the world of quadrilaterals, specifically focusing on kites. Kile word with diagonals \overline {od} od and \overline {wr} w r =\frac {1}} {2} \overline {wo}\cong \overline {wd} w. All right, kite word with diagnel's o d, w r, that's given w o equals w d and r o equals d property of a kite proper. Solution. Kite Word Has Diagonals Wr And Od.
From www.gauthmath.com
Solved Learning Activities Given Kite WORD with 1. Kite WORD with Kite Word Has Diagonals Wr And Od Sal proves that the diagonals of a kite are perpendicular, by using the sss and sas triangle. Let d1 and d2 be the lengths of the diagonals. Let wr be the perpendicular bisector of od. Kile word with diagonals \overline {od} od and \overline {wr} w r =\frac {1}} {2} \overline {wo}\cong \overline {wd} w. The video dives into the. Kite Word Has Diagonals Wr And Od.
From www.bartleby.com
Answered Theorem 10. In a kite, the… bartleby Kite Word Has Diagonals Wr And Od Solution for a kite word has diagonals wr and od. Kile word with diagonals \overline {od} od and \overline {wr} w r =\frac {1}} {2} \overline {wo}\cong \overline {wd} w. Kite word with diagonals overline wr and overline od prove: Let d1 and d2 be the lengths of the diagonals. Overline wr is the perpendicular bisector of overline od proof.. Kite Word Has Diagonals Wr And Od.
From quizlet.com
Prove that the diagonals of a kite are perpendicular to each Quizlet Kite Word Has Diagonals Wr And Od Solution for a kite word has diagonals wr and od. 1 recognize that word is a kite, which means the diagonals wr and od intersect at their midpoints 2 since word is a kite, the diagonals bisect. All right, kite word with diagnel's o d, w r, that's given w o equals w d and r o equals d property. Kite Word Has Diagonals Wr And Od.
From www.splashlearn.com
Properties of a Kite Definition, Diagonals, Examples, Facts Kite Word Has Diagonals Wr And Od Sal proves that the diagonals of a kite are perpendicular, by using the sss and sas triangle. Wr is the perpendicular bisector of od. Overline wr is the perpendicular bisector of overline od proof. Let d1 and d2 be the lengths of the diagonals. Kile word with diagonals \overline {od} od and \overline {wr} w r =\frac {1}} {2} \overline. Kite Word Has Diagonals Wr And Od.
From brainly.ph
Reasons(1Statements1. Kite WORD withdiagonals OD and WR.2. WO & WD and Kite Word Has Diagonals Wr And Od Kile word with diagonals \overline {od} od and \overline {wr} w r =\frac {1}} {2} \overline {wo}\cong \overline {wd} w. Let d1 and d2 be the lengths of the diagonals. Solution for a kite word has diagonals wr and od. Let wr be the perpendicular bisector of od. Sal proves that the diagonals of a kite are perpendicular, by using. Kite Word Has Diagonals Wr And Od.
From www.woojr.com
K is for Kite Word Tracing Woo! Jr. Kids Activities Children's Kite Word Has Diagonals Wr And Od Let wr be the perpendicular bisector of od. 1 recognize that word is a kite, which means the diagonals wr and od intersect at their midpoints 2 since word is a kite, the diagonals bisect. Sal proves that the diagonals of a kite are perpendicular, by using the sss and sas triangle. The video dives into the world of quadrilaterals,. Kite Word Has Diagonals Wr And Od.
From www.youtube.com
Prove Diagonals of a Kite are Perpendicular YouTube Kite Word Has Diagonals Wr And Od Solution for a kite word has diagonals wr and od. Kite word with diagonals overline wr and overline od prove: Overline wr is the perpendicular bisector of overline od proof. All right, kite word with diagnel's o d, w r, that's given w o equals w d and r o equals d property of a kite proper. Kile word with. Kite Word Has Diagonals Wr And Od.
From www.numerade.com
Prove that one diagonal of a kite bisects a pair of opposite angles and Kite Word Has Diagonals Wr And Od Sal proves that the diagonals of a kite are perpendicular, by using the sss and sas triangle. Let wr be the perpendicular bisector of od. All right, kite word with diagnel's o d, w r, that's given w o equals w d and r o equals d property of a kite proper. Kite word with diagonals overline wr and overline. Kite Word Has Diagonals Wr And Od.